Sine and Cosine Rules

This is the basics of the sine cos and tan graphs and how sine and cos relate to give you tan. It also shows how to differentiate sin and cos.

The output or range of both sine and cos is from -1 to 1 when given any angle. They can be shown on a graph where y = sin(x) and y = cos(x). In these graphs all the angles go along the x axis and you can see a wave type shape is formed

Sine Graph

Cosine Graph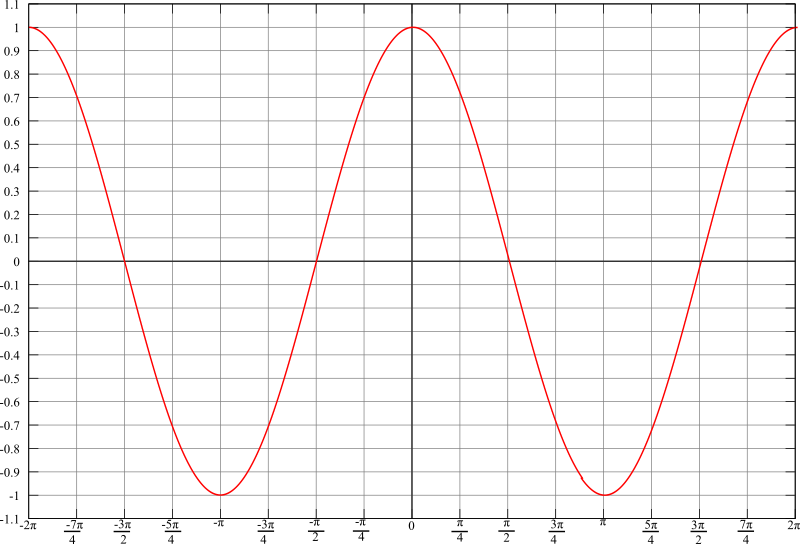

As you can see both the sin and cos graphs move periodically between -1 and 1 as the angles change, this pattern continues indefinitely because once you pass 360 degrees or 2 pi radians you will return back to the beginning. If you try to perform sin^-1 of a value out side the range -1 to 1 you will get an error.

Differentiate Sin and Cos

also notice that the gradient of the sin graph is the value of the cos graph for the same angle and that the gradient of the cos graph is the -value of the sin graph for that angle. This means that we can differentiate the sin and cos graphs:

if f(x) = sin(x) then f ‘ (x)=cos(x)

and

if f(x) = cos(x) then f ‘ (x) = -sin(x)

however if we use ax instead of x we must differentiate it by bringing the a out, when its just x this doesn’t matter as the differential of x is 1.

ie)

let y = sin(f(x))

now let u = f(x)

du/dx = f ‘ (x)

also

y=sin(u) as u = f(x)

dy/du = cos(u)

from the chain rule

dy/dx = du/dx * dy/du

therefore

if y = sin(f(x))

dy/dx = f ‘ (x)cos(f(x))

and similarly for cos

if y = cos(f(x))

dy/dx = -f ‘ (x)sin(f(x))